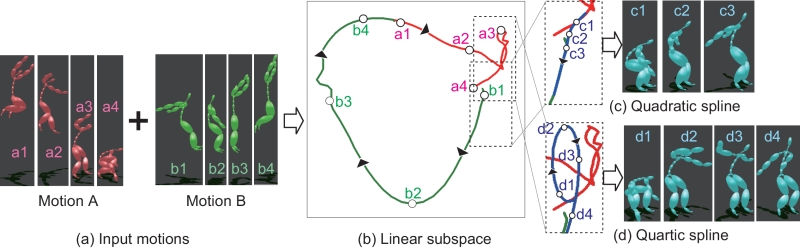
Spline Motion Transitions in Linear Subspaces

Posted on by

次元数削減法を応用したモーションデータ接続法の提案。接続元の2つのモーションデータを多次元尺度構成法を用いて次元削減し、それぞれ2次元潜在変数の軌跡として写像します。そして表示された軌跡を各種スプライン関数で接続し、もとのモーション空間に逆写像することで接続結果を得ます。スプライン関数の簡単な操作で、複雑なモーションの多様な接続バリエーションを生成できる点がポイントです。

We propose a system to concatenate motions with a smooth transition by applying a spline interpolation in a linear subspace in which each input motion is represented as a low-dimensional curve. By connecting the curves via a spline interpolation, a transition motion is synthesized according to a shape of the spline curve while preserving correlations among joints of input motions.

Publications